Tessellatable Numbers up to 2100, with corresponding Gaussian and Loeschian Number Pairs

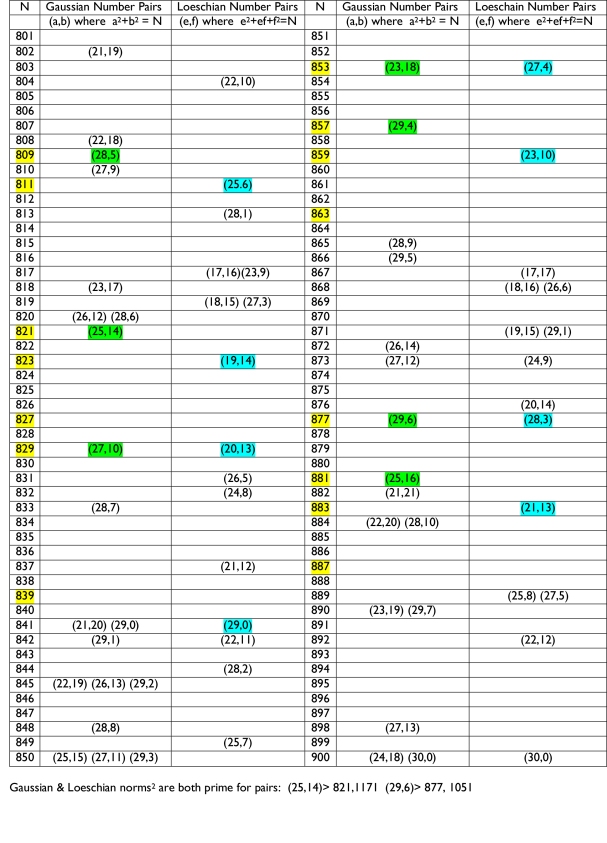
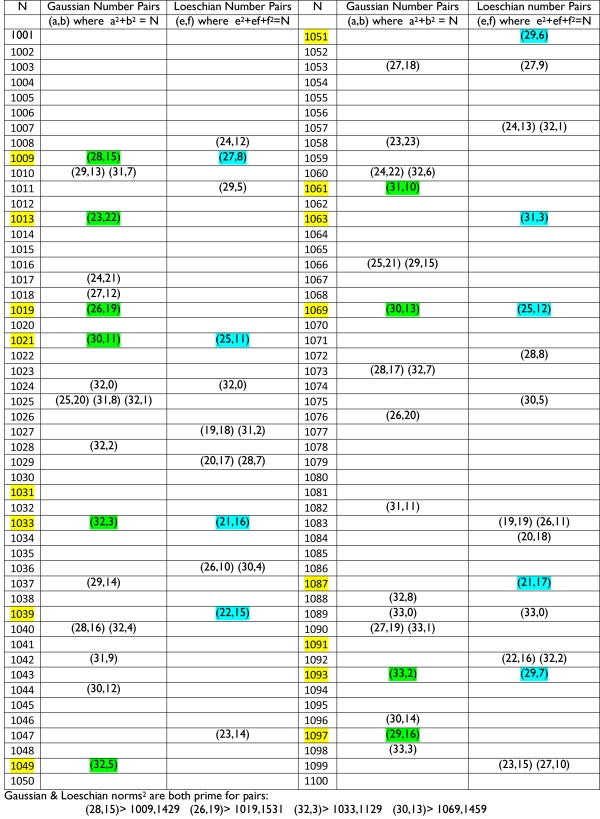
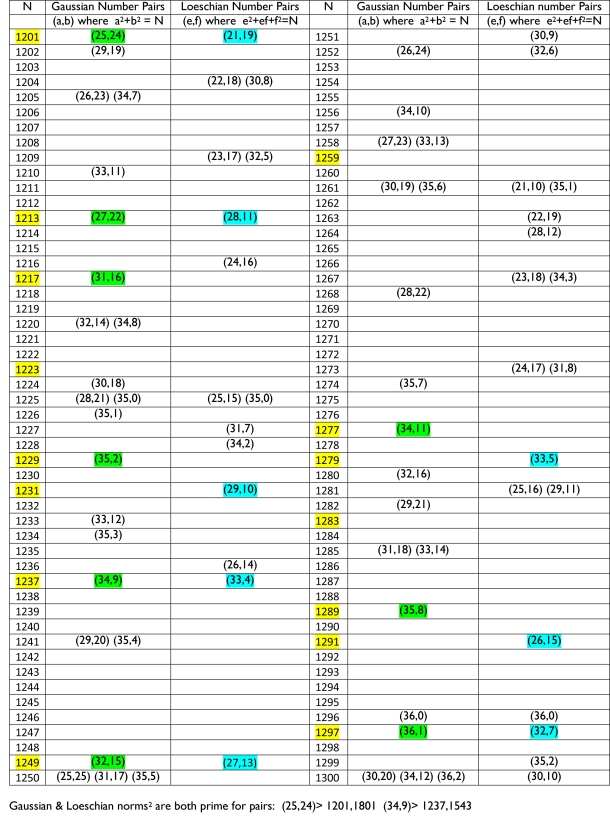
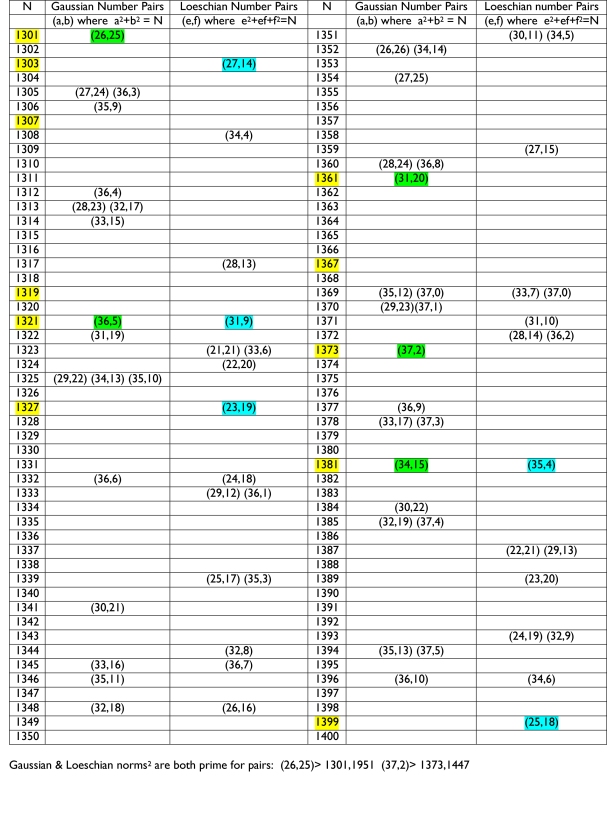
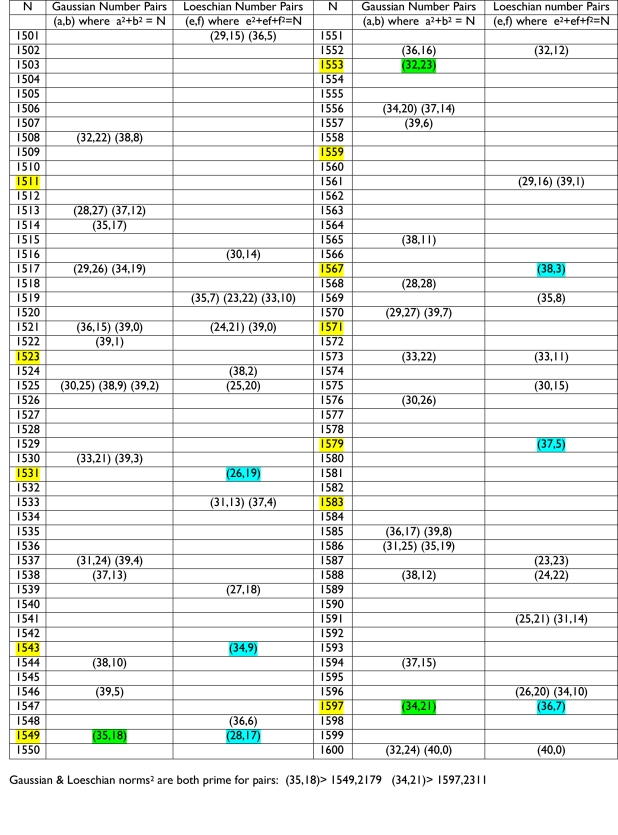
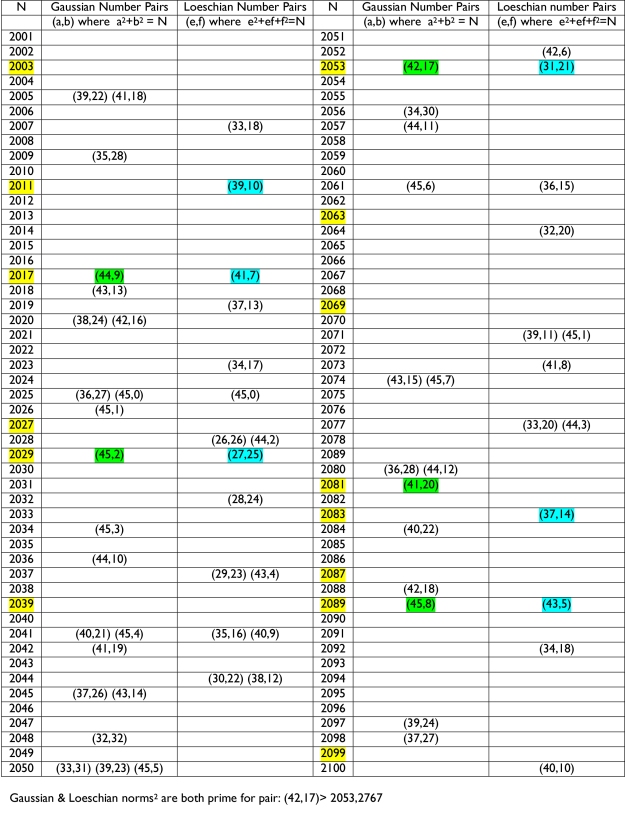
The following tables, which should appeal to your inner nerd and might even be USEFUL, pick out which integers N in the range 1 to 2100 are of the form a²+b² and/or of the form e²+ef+f². The first category are the square of the “norm” or “modulus” of Gaussian integers a+ib on the complex plane, the second the square of the norm of Loeschian integers e+ωf on the complex plane, where ω = (1+i√3)/2.

If N is of the form a²+b² it specifies a point (a,b) of the unit square lattice and corresponds to a tessellation on the square lattice. If N is of the form e²+ef+f² it specifies a point (e,f) of the triangular lattice and corresponds to a tessellation on the triangular lattice. This post replaces earlier tables of “tessellatable birth-years”, now deleted from the blog (though perhaps they live on in some limbonic Cloud).

Note that to every Loeschian number e²+ef+f² there corresponds an Eisenstein number g²-gh+h² with the same value of the norm, √N, but described by taking axes on the Argand diagram at 120° rather than 60° to each other. The Eisenstein representation I consider to be a menace; the negative sign in front of the product gh means that N jumps up and down as the value of  h increases. The Loeschian representation means that N always increases with an increase in either g or h.

In the tables, real primes are highlighted in yellow, Gaussian primes in green and Loeschian primes in blue.

There are all sorts of properties and patterns to be descried in these tables. Notice that there is one category of Gaussian and Loeschian primes for which N is NOT a real prime. For instance, N = 49 corresponds to Gaussian prime (7,0). this arise because                     N = 7 ≡ 3 modulo 4 and cannot be an a²+b² (which are always ≡ 0,1 or 2 modulo 4) and so corresponds to no Gaussian integer, and there is therefore no Gaussian integer that can be squared to give (7,0), so (7,0) is a Gaussian prime.

Similarly, N =25 corresponds to Loeschian prime (5,0) as N = 5 ≡ 2 modulo 3 and cannot be an e²+ef+f²  (which are always ≡ 0 or 1 modulo 3)so there is no Loeschian integer that can be squared to give (5,0).

Real PRIMES also cannot be ≡ 0 modulo 3 (divisible by 3),                                                 nor can they be ≡ 0 or 2 modulo 4 (divisible by 2 or 4 respectively), so all TESSALATABLE prime N are ≡ 1 modulo 4, corresponding to a Chinese Lattice Labyrinth or ≡ 1 modulo 3, corresponding to a Trefoil Lattice Labyrinth.

N = 121 corresponds to both Gaussian prime (11,0) and Loeschian prime (11,0) as       121 ≡  1 modulo 3 and 121 ≡ 1 modulo 4, that is 121 ≡  1 modulo 12.

My previous posts Eine kleine Mathmusik und Eine nicht so kleine Mathmusik  show how multiplying number pairs gives rise to tessellations of tessellations corresponding to the product(s) of the number pairs (Gaussian or Loeschian integers) and how Pythagorean triples can be generated. These triples, and other cases such as Gaussian  (9,2) and (7,6) both having N = 85 are a prominent feature of the tables. The lowest N corresponding to THREE Gaussian integers is 425, the lowest corresponding to FOUR is 1105. It is no coincidence that 5 is a factor of both these values of N and 13 a factor of the latter. The lowest N corresponding to three Loeschian integers is 931, the lowest corresponding to four is 1729. it is no coincidence that 7 is a factor of both these values of N and 13 a factor of the latter.

No value of N can correspond to more than a single prime Gaussian or Loeschian number. The fact that a real prime number can be an a²+b² in no more than one way was proved by Fermat. That it can be an e²+ef+f² ( or g²-gh+h²) in no more than one way appears also to be the case, though I haven’t come upon a formal proof. Note that this uniqueness extends to cases where N is not itself a prime but is the SQUARE of a prime which is not the square of the norm of a Gaussian or Loeschian integer, as outlined above.

Some real primes turn up in the intriguing and notorious adjacent pairs, such as 5 and 7, 29 and 31,……, 137 and 139 and so on without end. You will notice that these pairs correspond either to a Gaussian and a Loeschian integer respectively or the greater of the pair corresponds to both. This can be explained by simple algebra. Look for the cases where N ≡ 12 modulo 1.

Enough preambling. In the course of enhancing and playing with the  tables I have corrected some six “typos”, errors of transcription. Though I don’t like the admission, it’s “statistically likely” that a similar number of errors remains to be found. So just check the arithmetic before making use of an element of the tables and please comment on the blog post if you do spot an error.

Note that each Loeschian integer correspond to an Eisenstein integer; the number pair will of course be different in the two representations but the point on the complex plain referred to is the same and the value of N is the same. the conversion to Eisenstein (g,h) from Loeschian (e,f) is given by g = e+f, h = f. Another advantage of Loeschians; they are more economical with the natural numbers.

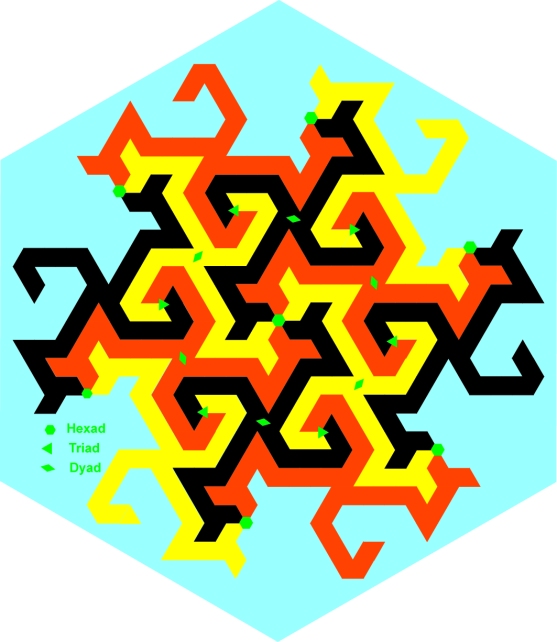
  To make this post a little less austere, here is a Lattice Labyrinth tessellation which is perhaps a comfortable compromise between  simply Escherable low-order and bewilderingly intricate higher-order examples, (7,4) in the Diamond family, which is to be found opposite N = 93 on the first page of the above table. (Note that Loeschian number (7,4) is not prime, but is the product of two prime Loeschians (1,1) and (5,1)) I’ve marked in green some of the axes of 6-fold, 3-fold and 2-fold rotational symmetry, which are arrayed in the same pattern as in a tessellation of the equilateral triangles of which each supertile is made up.

As always, here are some links to the inexpensive how-to-do-it  Lattice Labyrinths workbook which is available from the publisher or you-know-who , or from a good independent bookshop or via Google.